comments

difficulty

edit_url

rating

source

2642. 设计可以求最短路径的图类

题目描述

给你一个有 n 个节点的 有向带权 图，节点编号为 0 到 n - 1 。图中的初始边用数组 edges 表示，其中 edges[i] = [from_i, to_i, edgeCost_i] 表示从 from_i 到 to_i 有一条代价为 edgeCost_i 的边。

请你实现一个 Graph 类：

Graph(int n, int[][] edges) 初始化图有 n 个节点，并输入初始边。
addEdge(int[] edge) 向边集中添加一条边，其中 edge = [from, to, edgeCost] 。数据保证添加这条边之前对应的两个节点之间没有有向边。
int shortestPath(int node1, int node2) 返回从节点 node1 到 node2 的路径最小代价。如果路径不存在，返回 -1 。一条路径的代价是路径中所有边代价之和。

示例 1：

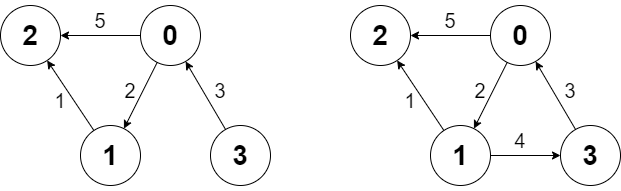
输入：
["Graph", "shortestPath", "shortestPath", "addEdge", "shortestPath"]
[[4, [[0, 2, 5], [0, 1, 2], [1, 2, 1], [3, 0, 3]]], [3, 2], [0, 3], [[1, 3, 4]], [0, 3]]
输出：
[null, 6, -1, null, 6]

解释：
Graph g = new Graph(4, [[0, 2, 5], [0, 1, 2], [1, 2, 1], [3, 0, 3]]);
g.shortestPath(3, 2); // 返回 6 。从 3 到 2 的最短路径如第一幅图所示：3 -> 0 -> 1 -> 2 ，总代价为 3 + 2 + 1 = 6 。
g.shortestPath(0, 3); // 返回 -1 。没有从 0 到 3 的路径。
g.addEdge([1, 3, 4]); // 添加一条节点 1 到节点 3 的边，得到第二幅图。
g.shortestPath(0, 3); // 返回 6 。从 0 到 3 的最短路径为 0 -> 1 -> 3 ，总代价为 2 + 4 = 6 。

提示：

1 <= n <= 100
0 <= edges.length <= n * (n - 1)
edges[i].length == edge.length == 3
0 <= from_i, to_i, from, to, node1, node2 <= n - 1
1 <= edgeCost_i, edgeCost <= 10⁶
图中任何时候都不会有重边和自环。
调用 addEdge 至多 100 次。
调用 shortestPath 至多 100 次。

解法

方法一：Dijsktra 算法

在初始化函数中，我们先用邻接矩阵 $g$ 存储图的边权，其中 $g_{ij}$ 表示从节点 $i$ 到节点 $j$ 的边权，如果 $i$ 和 $j$ 之间没有边，则 $g_{ij}$ 的值为 $\infty$。

在 addEdge 函数中，我们更新 $g_{ij}$ 的值为 $edge[2]$。

在 shortestPath 函数中，我们使用 Dijsktra 算法求从节点 $node1$ 到节点 $node2$ 的最短路径，其中 $dist[i]$ 表示从节点 $node1$ 到节点 $i$ 的最短路径，$vis[i]$ 表示节点 $i$ 是否已经被访问过。我们初始化 $dist[node1]$ 为 $0$，其余的 $dist[i]$ 均为 $\infty$。然后我们遍历 $n$ 次，每次找到当前未被访问过的节点 $t$，使得 $dist[t]$ 最小。然后我们将节点 $t$ 标记为已访问，然后更新 $dist[i]$ 的值为 $min(dist[i], dist[t] + g_{ti})$。最后我们返回 $dist[node2]$，如果 $dist[node2]$ 为 $\infty$，则说明从节点 $node1$ 到节点 $node2$ 不存在路径，返回 $-1$。

时间复杂度 $O(n^2 \times q)$，空间复杂度 $O(n^2)$。其中 $n$ 为节点数，而 $q$ 为 shortestPath 函数的调用次数。

Python3

class Graph:
    def __init__(self, n: int, edges: List[List[int]]):
        self.n = n
        self.g = [[inf] * n for _ in range(n)]
        for f, t, c in edges:
            self.g[f][t] = c

    def addEdge(self, edge: List[int]) -> None:
        f, t, c = edge
        self.g[f][t] = c

    def shortestPath(self, node1: int, node2: int) -> int:
        dist = [inf] * self.n
        dist[node1] = 0
        vis = [False] * self.n
        for _ in range(self.n):
            t = -1
            for j in range(self.n):
                if not vis[j] and (t == -1 or dist[t] > dist[j]):
                    t = j
            vis[t] = True
            for j in range(self.n):
                dist[j] = min(dist[j], dist[t] + self.g[t][j])
        return -1 if dist[node2] == inf else dist[node2]


# Your Graph object will be instantiated and called as such:
# obj = Graph(n, edges)
# obj.addEdge(edge)
# param_2 = obj.shortestPath(node1,node2)

Java

class Graph {
    private int n;
    private int[][] g;
    private final int inf = 1 << 29;

    public Graph(int n, int[][] edges) {
        this.n = n;
        g = new int[n][n];
        for (var f : g) {
            Arrays.fill(f, inf);
        }
        for (int[] e : edges) {
            int f = e[0], t = e[1], c = e[2];
            g[f][t] = c;
        }
    }

    public void addEdge(int[] edge) {
        int f = edge[0], t = edge[1], c = edge[2];
        g[f][t] = c;
    }

    public int shortestPath(int node1, int node2) {
        int[] dist = new int[n];
        boolean[] vis = new boolean[n];
        Arrays.fill(dist, inf);
        dist[node1] = 0;
        for (int i = 0; i < n; ++i) {
            int t = -1;
            for (int j = 0; j < n; ++j) {
                if (!vis[j] && (t == -1 || dist[t] > dist[j])) {
                    t = j;
                }
            }
            vis[t] = true;
            for (int j = 0; j < n; ++j) {
                dist[j] = Math.min(dist[j], dist[t] + g[t][j]);
            }
        }
        return dist[node2] >= inf ? -1 : dist[node2];
    }
}

/**
 * Your Graph object will be instantiated and called as such:
 * Graph obj = new Graph(n, edges);
 * obj.addEdge(edge);
 * int param_2 = obj.shortestPath(node1,node2);
 */

C++

class Graph {
public:
    Graph(int n, vector<vector<int>>& edges) {
        this->n = n;
        g = vector<vector<int>>(n, vector<int>(n, inf));
        for (auto& e : edges) {
            int f = e[0], t = e[1], c = e[2];
            g[f][t] = c;
        }
    }

    void addEdge(vector<int> edge) {
        int f = edge[0], t = edge[1], c = edge[2];
        g[f][t] = c;
    }

    int shortestPath(int node1, int node2) {
        vector<bool> vis(n);
        vector<int> dist(n, inf);
        dist[node1] = 0;
        for (int i = 0; i < n; ++i) {
            int t = -1;
            for (int j = 0; j < n; ++j) {
                if (!vis[j] && (t == -1 || dist[t] > dist[j])) {
                    t = j;
                }
            }
            vis[t] = true;
            for (int j = 0; j < n; ++j) {
                dist[j] = min(dist[j], dist[t] + g[t][j]);
            }
        }
        return dist[node2] >= inf ? -1 : dist[node2];
    }

private:
    vector<vector<int>> g;
    int n;
    const int inf = 1 << 29;
};

/**
 * Your Graph object will be instantiated and called as such:
 * Graph* obj = new Graph(n, edges);
 * obj->addEdge(edge);
 * int param_2 = obj->shortestPath(node1,node2);
 */

Go

const inf = 1 << 29

type Graph struct {
	g [][]int
}

func Constructor(n int, edges [][]int) Graph {
	g := make([][]int, n)
	for i := range g {
		g[i] = make([]int, n)
		for j := range g[i] {
			g[i][j] = inf
		}
	}
	for _, e := range edges {
		f, t, c := e[0], e[1], e[2]
		g[f][t] = c
	}
	return Graph{g}
}

func (this *Graph) AddEdge(edge []int) {
	f, t, c := edge[0], edge[1], edge[2]
	this.g[f][t] = c
}

func (this *Graph) ShortestPath(node1 int, node2 int) int {
	n := len(this.g)
	dist := make([]int, n)
	for i := range dist {
		dist[i] = inf
	}
	vis := make([]bool, n)
	dist[node1] = 0
	for i := 0; i < n; i++ {
		t := -1
		for j := 0; j < n; j++ {
			if !vis[j] && (t == -1 || dist[t] > dist[j]) {
				t = j
			}
		}
		vis[t] = true
		for j := 0; j < n; j++ {
			dist[j] = min(dist[j], dist[t]+this.g[t][j])
		}
	}
	if dist[node2] >= inf {
		return -1
	}
	return dist[node2]
}

/**
 * Your Graph object will be instantiated and called as such:
 * obj := Constructor(n, edges);
 * obj.AddEdge(edge);
 * param_2 := obj.ShortestPath(node1,node2);
 */

TypeScript

class Graph {
    private g: number[][] = [];
    private inf: number = 1 << 29;

    constructor(n: number, edges: number[][]) {
        this.g = Array.from({ length: n }, () => Array(n).fill(this.inf));
        for (const [f, t, c] of edges) {
            this.g[f][t] = c;
        }
    }

    addEdge(edge: number[]): void {
        const [f, t, c] = edge;
        this.g[f][t] = c;
    }

    shortestPath(node1: number, node2: number): number {
        const n = this.g.length;
        const dist: number[] = new Array(n).fill(this.inf);
        dist[node1] = 0;
        const vis: boolean[] = new Array(n).fill(false);
        for (let i = 0; i < n; ++i) {
            let t = -1;
            for (let j = 0; j < n; ++j) {
                if (!vis[j] && (t === -1 || dist[j] < dist[t])) {
                    t = j;
                }
            }
            vis[t] = true;
            for (let j = 0; j < n; ++j) {
                dist[j] = Math.min(dist[j], dist[t] + this.g[t][j]);
            }
        }
        return dist[node2] >= this.inf ? -1 : dist[node2];
    }
}

/**
 * Your Graph object will be instantiated and called as such:
 * var obj = new Graph(n, edges)
 * obj.addEdge(edge)
 * var param_2 = obj.shortestPath(node1,node2)
 */

C#

public class Graph {
    private int n;
    private int[,] g;
    private readonly int inf = 1 << 29;

    public Graph(int n, int[][] edges) {
        this.n = n;
        g = new int[n, n];
        for (int i = 0; i < n; i++) {
            for (int j = 0; j < n; j++) {
                g[i, j] = inf;
            }
        }
        foreach (var e in edges) {
            int f = e[0], t = e[1], c = e[2];
            g[f, t] = c;
        }
    }

    public void AddEdge(int[] edge) {
        int f = edge[0], t = edge[1], c = edge[2];
        g[f, t] = c;
    }

    public int ShortestPath(int node1, int node2) {
        int[] dist = new int[n];
        bool[] vis = new bool[n];
        Array.Fill(dist, inf);
        dist[node1] = 0;
        for (int i = 0; i < n; ++i) {
            int t = -1;
            for (int j = 0; j < n; ++j) {
                if (!vis[j] && (t == -1 || dist[t] > dist[j])) {
                    t = j;
                }
            }
            vis[t] = true;
            for (int j = 0; j < n; ++j) {
                dist[j] = Math.Min(dist[j], dist[t] + g[t, j]);
            }
        }
        return dist[node2] >= inf ? -1 : dist[node2];
    }
}

/**
 * Your Graph object will be instantiated and called as such:
 * Graph obj = new Graph(n, edges);
 * obj.AddEdge(edge);
 * int param_2 = obj.ShortestPath(node1,node2);
 */

Provide feedback

Saved searches

Use saved searches to filter your results more quickly

README.md

README.md

2642. 设计可以求最短路径的图类

题目描述

解法

方法一：Dijsktra 算法

Python3

Java

C++

Go

TypeScript

C#

Files

README.md

Latest commit

History

README.md

File metadata and controls

2642. 设计可以求最短路径的图类

题目描述

解法

方法一：Dijsktra 算法

Python3

Java

C++

Go

TypeScript

C#