README_EN.md

comments	difficulty	edit_url	rating	source	tags
true	Hard	https://github.com/doocs/leetcode/edit/main/solution/2500-2599/2508.Add%20Edges%20to%20Make%20Degrees%20of%20All%20Nodes%20Even/README_EN.md	2060	Weekly Contest 324 Q3	Graph Hash Table

2508. Add Edges to Make Degrees of All Nodes Even

中文文档

Description

There is an undirected graph consisting of n nodes numbered from 1 to n. You are given the integer n and a 2D array edges where edges[i] = [ai, bi] indicates that there is an edge between nodes ai and bi. The graph can be disconnected.

You can add at most two additional edges (possibly none) to this graph so that there are no repeated edges and no self-loops.

Return true if it is possible to make the degree of each node in the graph even, otherwise return false.

The degree of a node is the number of edges connected to it.

 

Example 1:

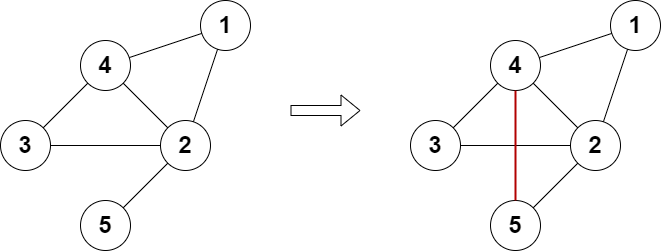
Input: n = 5, edges = [[1,2],[2,3],[3,4],[4,2],[1,4],[2,5]]
Output: true
Explanation: The above diagram shows a valid way of adding an edge.
Every node in the resulting graph is connected to an even number of edges.

Example 2:

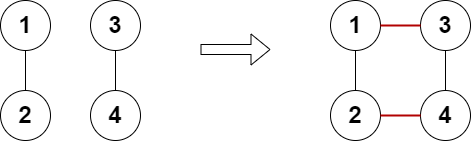
Input: n = 4, edges = [[1,2],[3,4]]
Output: true
Explanation: The above diagram shows a valid way of adding two edges.

Example 3:

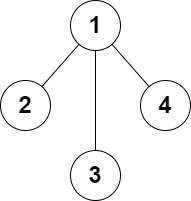
Input: n = 4, edges = [[1,2],[1,3],[1,4]]
Output: false
Explanation: It is not possible to obtain a valid graph with adding at most 2 edges.

 

Constraints:

• 3 <= n <= 105
• 2 <= edges.length <= 105
• edges[i].length == 2
• 1 <= ai, bi <= n
• ai != bi
• There are no repeated edges.

Solutions

Solution 1

Python3

class Solution:
    def isPossible(self, n: int, edges: List[List[int]]) -> bool:
        g = defaultdict(set)
        for a, b in edges:
            g[a].add(b)
            g[b].add(a)
        vs = [i for i, v in g.items() if len(v) & 1]
        if len(vs) == 0:
            return True
        if len(vs) == 2:
            a, b = vs
            if a not in g[b]:
                return True
            return any(a not in g[c] and c not in g[b] for c in range(1, n + 1))
        if len(vs) == 4:
            a, b, c, d = vs
            if a not in g[b] and c not in g[d]:
                return True
            if a not in g[c] and b not in g[d]:
                return True
            if a not in g[d] and b not in g[c]:
                return True
            return False
        return False

Java

class Solution {
    public boolean isPossible(int n, List<List<Integer>> edges) {
        Set<Integer>[] g = new Set[n + 1];
        Arrays.setAll(g, k -> new HashSet<>());
        for (var e : edges) {
            int a = e.get(0), b = e.get(1);
            g[a].add(b);
            g[b].add(a);
        }
        List<Integer> vs = new ArrayList<>();
        for (int i = 1; i <= n; ++i) {
            if (g[i].size() % 2 == 1) {
                vs.add(i);
            }
        }
        if (vs.size() == 0) {
            return true;
        }
        if (vs.size() == 2) {
            int a = vs.get(0), b = vs.get(1);
            if (!g[a].contains(b)) {
                return true;
            }
            for (int c = 1; c <= n; ++c) {
                if (a != c && b != c && !g[a].contains(c) && !g[c].contains(b)) {
                    return true;
                }
            }
            return false;
        }
        if (vs.size() == 4) {
            int a = vs.get(0), b = vs.get(1), c = vs.get(2), d = vs.get(3);
            if (!g[a].contains(b) && !g[c].contains(d)) {
                return true;
            }
            if (!g[a].contains(c) && !g[b].contains(d)) {
                return true;
            }
            if (!g[a].contains(d) && !g[b].contains(c)) {
                return true;
            }
            return false;
        }
        return false;
    }
}

C++

class Solution {
public:
    bool isPossible(int n, vector<vector<int>>& edges) {
        vector<unordered_set<int>> g(n + 1);
        for (auto& e : edges) {
            int a = e[0], b = e[1];
            g[a].insert(b);
            g[b].insert(a);
        }
        vector<int> vs;
        for (int i = 1; i <= n; ++i) {
            if (g[i].size() % 2) {
                vs.emplace_back(i);
            }
        }
        if (vs.size() == 0) {
            return true;
        }
        if (vs.size() == 2) {
            int a = vs[0], b = vs[1];
            if (!g[a].count(b)) return true;
            for (int c = 1; c <= n; ++c) {
                if (a != b && b != c && !g[a].count(c) && !g[c].count(b)) {
                    return true;
                }
            }
            return false;
        }
        if (vs.size() == 4) {
            int a = vs[0], b = vs[1], c = vs[2], d = vs[3];
            if (!g[a].count(b) && !g[c].count(d)) return true;
            if (!g[a].count(c) && !g[b].count(d)) return true;
            if (!g[a].count(d) && !g[b].count(c)) return true;
            return false;
        }
        return false;
    }
};

Go

func isPossible(n int, edges [][]int) bool {
	g := make([]map[int]bool, n+1)
	for _, e := range edges {
		a, b := e[0], e[1]
		if g[a] == nil {
			g[a] = map[int]bool{}
		}
		if g[b] == nil {
			g[b] = map[int]bool{}
		}
		g[a][b], g[b][a] = true, true
	}
	vs := []int{}
	for i := 1; i <= n; i++ {
		if len(g[i])%2 == 1 {
			vs = append(vs, i)
		}
	}
	if len(vs) == 0 {
		return true
	}
	if len(vs) == 2 {
		a, b := vs[0], vs[1]
		if !g[a][b] {
			return true
		}
		for c := 1; c <= n; c++ {
			if a != c && b != c && !g[a][c] && !g[c][b] {
				return true
			}
		}
		return false
	}
	if len(vs) == 4 {
		a, b, c, d := vs[0], vs[1], vs[2], vs[3]
		if !g[a][b] && !g[c][d] {
			return true
		}
		if !g[a][c] && !g[b][d] {
			return true
		}
		if !g[a][d] && !g[b][c] {
			return true
		}
		return false
	}
	return false
}